Richard AOUN, Stationary probability measures on the projective space
Summary
Let (X_i) be a sequence of iid GL_d(R)-valued random matrices (where the dimension d is being fixed once for all). The (non commutative) random walk S_n=X_n ... X_1 on the group GL_d(R) induces a Markov chain on the projective space P(R^d). The goal of the talk is to give a full (qualitative) classification of the stationary probability measures of this Markov chain without any irreducibility assumption, generalizing results of Bougerol--Picard (92) concerning affine random walks and linking the works of Furstenberg--Kifer (82) and those Guivarch--Raugi (07) and Benoist--Quint (16) in the reductive case. Joint work with Çağri Sert.
Julien BRÉMONT, Random walk in a stratified quasi-periodic environment in the plane
Summary
We discuss the recurrence properties of the model indicated in the title. The analysis involves former results of Jean-Pierre Conze and Yves Guivarc'h (2000) on random walks in random medium and quasi-invariant measures, that we shall recall precisely.
Sara BROFFERIO, Random walks on semigroup of matrices: the case of rank one matrices
Summary
Random products of matrices have been widely studied in the last years for both
their theoretical interest and various application. We now have a rich and complete
theory for random walks on linear group, however less is known when the matrices are
not supposed to be invertible.
The goal of this talk is to present through the exemple of the semigroup of rank one
matrices the interest of exploring random walks on semigroups, presenting
reasonable generalisation of the group case, new challenges and some results.
Guy COHEN, On the empirical process sampled along a stationary process
Summary
Nguyen-Thi DANG Equidistribution of periodic tori
Summary
Bowen and Margulis independently proved in the 70s that closed geodesics on compact hyperbolic surfaces equidistribute towards the measure of maximal entropy. From a homogeneous dynamics
point of view, this measure is the quotient of the Haar measure on PSL(2,R) modulo some discrete
cocompact sugroup.
In a joint work with Jialun Li, we investigate the higher rank setting of this problem by taking a
higher rank Lie group (like SL(d,R) for d>2) and by studying the dynamical properties of geodesic
flows in higher rank : the so-called Weyl chamber flows and their induced diagonal action. We obtain an
equidistribution formula of periodic tori (instead of closed orbits of the geodesic flow).
Jérôme DEDECKER Mixing properties of a class of dynamical systems
Summary
We compute the tau-mixing coefficients of a class of dynamical systems. We give some applications
to deviations inequalities in case of polynomial or sub-exponential decay of
correlations. Based on a joint work with C. Cuny and F. Merlevède
Kasun FERNANDO, Limit Theorems for a class of unbounded observables with an
application to "Sampling the Lindelöf hypothesis"
Summary
We prove the Central Limit Theorem (CLT), the first order Edgeworth Expansion and a
Mixing Local Central Limit Theorem (MLCLT) for Birkhoff sums of a class of unbounded
heavily oscillating observables over a family of full-branch piecewise smooth expanding
maps of the interval. As a corollary, we obtain the corresponding results for
Boolean-type transformations on the real line. The class of observables in the CLT and
the MLCLT on the real line include the real part, the imaginary part and the absolute
value of the Riemann zeta function. Thus obtained CLT and MLCLT for the Riemann zeta
function are in the spirit of the results of Lifschitz & Weber (2009) and Steuding
(2012) who have proven the Strong Law of Large Numbers for sampling the Lindelöf
hypothesis.
Krzysztof FRĄCZEK, Deviation Spectrum of Ergodic Integrals for Locally Hamiltonian Flows on Surfaces
Summary
The talk will consists of a long historical introduction to the topic of deviation
of ergodic averages for locally Hamiltonian flows on compact surafces as well as
some current results obtained in collaboration with Corinna Ulcigrai and Minsung Kim.
New developments include a better understanding of the asymptotic of so-called error
term (in non-degenerate regime) and the appearance of new exponents in the deviation
spectrum (in degenerate regime).
Stefano GALATOLO, Self Consistent Transfer Operators in a Weak and Not So
Weak Coupling Regime. Invariant Measures, Convergence to Equilibrium, Linear Response
Summary
We describe a general approach to the theory of self consistent transfer operators. These operators have been introduced as tools for the study of the statistical properties of a large number of all to all interacting dynamical systems subjected to a mean field coupling.
We consider a large class of self consistent transfer operators and prove general statements about existence of invariant measures, speed of convergence to equilibrium, statistical stability and linear response.
While most of the results presented are valid in a weak coupling regime, the existence results for the invariant measures we show also hold outside the weak coupling regime.
We also consider the problem of finding the optimal coupling between maps in order to change the statistical properties of the system in a prescribed way.
Sakshi JAIN On dynamics of non-Markov systems
Summary
We study transfer operators associated to piecewise monotone interval transformations
and show that the essential spectrum is large whenever the Banach space bounds
L^\infty and the transformation fails to be Markov.
Constructing a family of Banach spaces we show that the lower bound on the
essential spectral radius is optimal. Indeed, these Banach spaces realise an
essential spectral radius as close as desired to be the theoretical best possible case.
Emmanuel LESIGNE
Carlos MATHEUS SILVA, Non-conical strictly convex divisible sets are highly anisotropic
Summary
Strictly convex divisible sets X are the universal covers of real projective compact
manifolds M. From the dynamical point of view, these objects yield natural examples
of topologically mixing Anosov flows. The boundary of a strictly convex divisible set
is not very smooth in general: unless X is conical, i.e., an ellipsoid (and, thus,
M is a hyperbolic manifold), its boundary is not C^2. In this talk, based on joint
work with P. Foulon and P. Hubert, we shall discuss the infinitesimal bends at a
typical point of the boundary of a non-conical strictly convex divisible set: in
particular, we shall see that these infinitesimal bends are highly anisotropic
(i.e., they are distinct in distinct directions) thanks to the features of certain
locally constant cocycles over uniformly hyperbolic systems.
Ian MELBOURNE, Interpretation of stochastic integrals, and the Levy area
Summary
An important question in stochastic analysis is the appropriate interpretation of
stochastic integrals. The classical Wong-Zakai theorem gives sufficient conditions
under which smooth integrals converge to Stratonovich stochastic integrals. The
conditions are automatic in one-dimension, but in higher dimensions it is necessary
to take account of corrections stemming from the Levy area.
In previous work with Kelly, we justified the Levy area correction for large classes of
deterministic systems, bypassing any stochastic modelling assumptions.
This talk addresses a much less studied question: is the Levy area zero or nonzero for
systems of physical interest, eg Hamiltonian time-reversible systems? In recent work
with Gottwald, we classify (and clarify) the situations where such structure forces the
Levy area to vanish. Outside of these rare situations, we show that the Levy area is
typically nonzero.
Jean-François QUINT, Local limit theorems for conditioned Birkhoff sums
Summary
For a centered random walk S_n on R, given x in R, one can estimate
the probability that x+S_n belongs to an interval [a,b], while all the x+S_k, 0< k< n,
have remained non-negative: it decays as n^{-3/2}.
Following a general principle which one can date back to Sinai, this should
have an analogue for Birkhoff sums of Hölder continuous observables over
hyperbolic dynamical systems. Indeed, this is the case, as follows from our joint
work with Ion Grama and Hui Xiao.
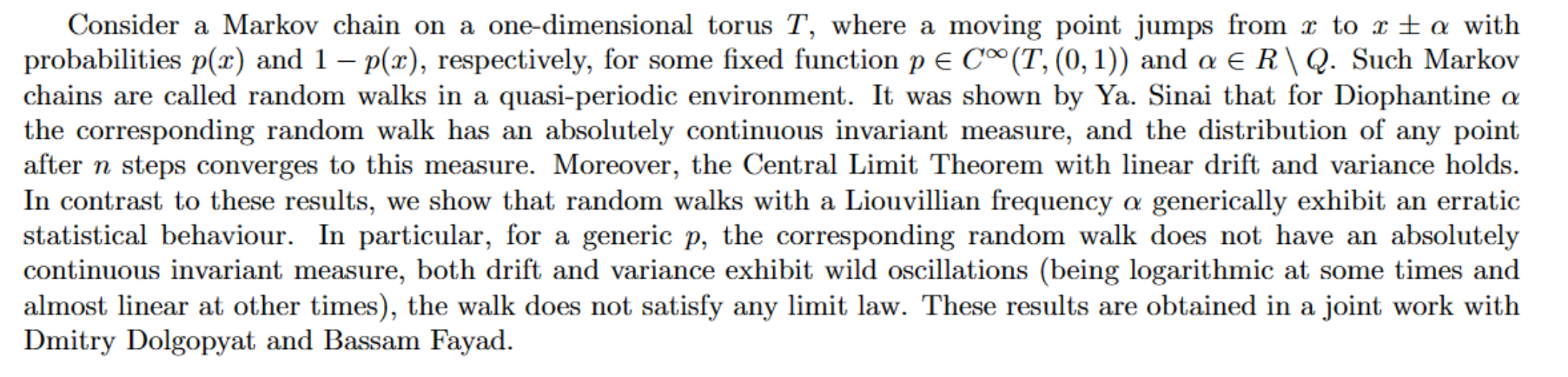
Maria SAPRYKINA, Erratic behaviour for one-dimensional random walks in a generic quasi-periodic environment
Summary
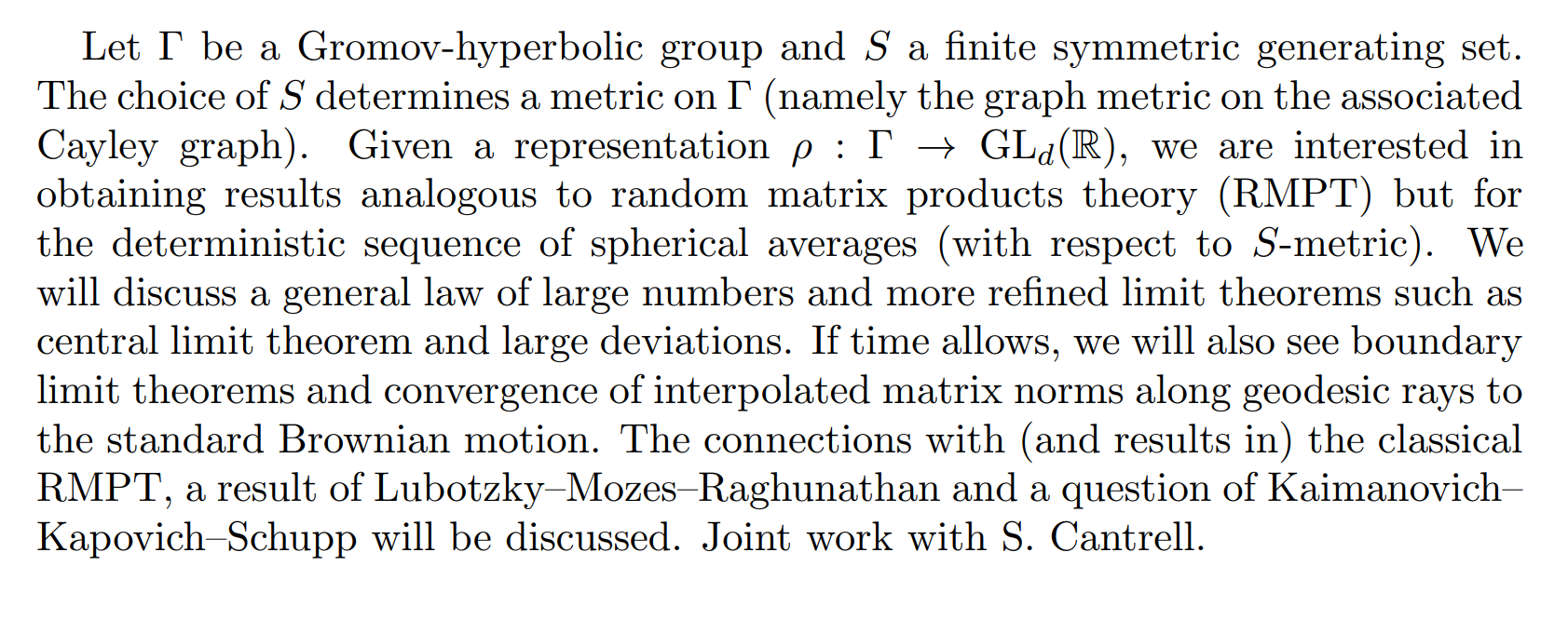
Çağri SERT, Counting limit theorems for representations of Gromov-hyperbolic
groups
Summary
Julien TRÉVISAN, Limit laws in the lattice point problem
Summary
In this talk, we are going to take interest in the following general problem.
Let L be a unimodular lattice of R^{d}. Let S be a measurable set of finite volume.
What is the number N(S,L) of points that belong to both L and S? When S is
sufficiently regular, it can be shown that this number N(S,L) is approximated by
vol(S) to within an error denoted by R(S,L). The aim of this talk is to present
several results that gives us a better idea of the behaviour of R(S,L) in different
situations: for example, when S is the united disk dilated by a factor t with t going
to infinity and L being a random unimodular lattice or when S is a parallelogram
dilated by a factor t with t going to infinity and L being a random unimodular lattice.
Polina VYTNOVA, A new approach to compute Lyapunov exponents
Hong-Kun ZHANG, Hyperbolicity and entropy of convex billiards
Summary
Billiards are well-known models first introduced by Birkhoff as paradigmatic examples
of Hamiltonian systems, and pioneered by Yakov Sinai, Leonid Bunimovich, Nikolai
Chernov, etc., as a mathematical model for the Lorenz systems and hard-ball gases.
Since then billiards have acquired increasing importance as they shed light in
understanding thermodynamic limits, connected to deep issues in quantum and wave
physics all the way to quantum chaos. However for some Bunimovich billiards, whose
boundary consists of only arcs and straight lines, even the hyperbolicity is not known,
not to mention ergodicity and other chaotic properties. In this talk, I will discuss
these new classes of convex billiards and their properties. The hyperbolicity for some
of them is only numerically proved, which leaves many open questions to explore. I
will also review my recent collaboration work with Michal Misiurewicz about topological
entropy for Bunimovich stadium.